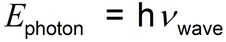Module 2202
Light: wave-particle “duality”
Sometimes it seems to us that light is a wave, and sometimes it seems to be a stream of particles. What's going on?
What is light? Waves, particles, or both, or neither?
"Dual nature" of light?
Or, dual nature of our sense-making?
Why might we refer the 'dual nature' of light (with quotation marks), but the dual nature of our sense-making without quotation marks)? Prof Bob and Aussie try to sort it out ........
Aha! So we should re-word the title on Prof Bob's board? To ...... ?
KEY IDEAS - Light: wave-particle 'duality'
Scientists in the early 1900 contemplating the nature of light (rather than their navels). What a mystery! Can you pick out Einstein in this group?
What duality?
Scientists have discussed and argued about the nature of light for centuries: that is, they have debated the question “What is light?”
The nature of light is very complex, even mysterious, and seems to be different from everything else that we experience in our everyday macro world.
So scientists resort to explaining its behaviour in two different ways, depending on the circumstances – in some situations light behaves in ways that are best explainable by considering it to be a waveform, and in other situations its behaviour only makes sense to us as movement of particles.
Because of this, scientists frequently refer to the “dual nature” of light.
This does not mean that a ray of light light is a particle and a wave. Nor that it is a particle or a wave. And certainly not that it is sometimes a particle and sometimes a wave, depending on the situation.
In fact, light surely does not have a dual nature: it is what it is.
But because of our inability to understand what it is, we resort to two different models taken from phenomena in our common experience – waves and particles.
So a better label would be the dual nature of our explanations. And then there is no need for quotation marks (around dual nature) because this duality is real.
The nature of light is very complex, even mysterious, and seems to be different from everything else that we experience in our everyday macro world.
So scientists resort to explaining its behaviour in two different ways, depending on the circumstances – in some situations light behaves in ways that are best explainable by considering it to be a waveform, and in other situations its behaviour only makes sense to us as movement of particles.
Because of this, scientists frequently refer to the “dual nature” of light.
This does not mean that a ray of light light is a particle and a wave. Nor that it is a particle or a wave. And certainly not that it is sometimes a particle and sometimes a wave, depending on the situation.
In fact, light surely does not have a dual nature: it is what it is.
But because of our inability to understand what it is, we resort to two different models taken from phenomena in our common experience – waves and particles.
So a better label would be the dual nature of our explanations. And then there is no need for quotation marks (around dual nature) because this duality is real.
How did we come to this?
In the 1600s, competing theories about the nature of light were pushed by Christiaan Huygens (waveforms) and Isaac Newton (particles).
The wave model of light dominated for a couple of centuries. It successfully accounts, even quantitatively, for the phenomena of reflection, refraction, diffraction at slits and interference patterns.
But in the early 1900s, the photoelectric effect was observed. Ultraviolet light shone on the surface of certain materials gave rise to the ejection of electrons, the energy of which could be measured.
The photoelectric effect is not consistent with light being a waveform, and Einstein proposed that light is not a wave, but a collection of “packets” of energy – which we call photons.
Crucial experiments were conducted by American physicist Arthur Compton who made measurements on the interaction between light and electrons.
He showed that electrons set in motion by the action of a light ray behaved exactly as if they had been struck by a pulse of energy corresponding with Einstein’s relationship – rather than a continuous energy source.
Energy equivalence of a wave and a photon
Regardless of what light is, we can calculate the relationship between the energy of a photon (if we consider it to be a photon) and the frequency of the wave (if we consider it to be a wave).
Einstein deduced that the energy of the photon (E) is proportional to the frequency (ν) of the wave. This can be expressed as:
in which the proportionality constant, h, is called the Planck constant.
The accepted value of the Planck constant is 6.626 × 10-34 J s.
The accepted value of the Planck constant is 6.626 × 10-34 J s.
We can use this formula to calculate the energy of a photon from either the frequency or wavelength of the light.
For example, we see light with wavelengths in the range 650 nm to 720 nm as various hues of red. Let’s, just for example, consider light with wavelength λ = 700 nm.
From this wavelength and the speed of light (c), we can calculate the frequency of the waveform (ν), and then use that to calculate the energy of the corresponding photons (E):
For example, we see light with wavelengths in the range 650 nm to 720 nm as various hues of red. Let’s, just for example, consider light with wavelength λ = 700 nm.
From this wavelength and the speed of light (c), we can calculate the frequency of the waveform (ν), and then use that to calculate the energy of the corresponding photons (E):
Photons with this energy are absorbed from white light if the energy gap between “allowed” energy levels of electrons in the absorbing species is exactly this value. The transmitted light will be blue-green.
Elephant waves
We don’t usually think of elephants as waves, do we? Or even mosquitoes. Or planets. But everything that has been said above, and the photon-wave energy calculation, is equally applicable to all things that we normally think about as objects, and all things that we normally think about as waves.
The ‘crossover’ region where it is sensible to consider both the wave form and photon form of the same phenomenon are approximately from electrons (on the high energy side) to x-rays (on the low energy side).
In principle, you can calculate your own wavelength when walking at a certain speed. Now there is a challenge!
The ‘crossover’ region where it is sensible to consider both the wave form and photon form of the same phenomenon are approximately from electrons (on the high energy side) to x-rays (on the low energy side).
In principle, you can calculate your own wavelength when walking at a certain speed. Now there is a challenge!
And just imagine .......
The Planck constant is a so-called “universal constant” – unchangeable throughout the universe, like the speed of light, or the gravitational constant: not a value decided by a scientific world authority, but something we are lumbered with. Don’t drive yourself mad wondering why its value should be so ……
But just suppose that we were in a universe where the value of the Planck constant were far different. Then perhaps the perceived “wave-particle duality” might be applicable to human beings!? Imagine seeing Aunt Mary being diffracted as she approaches a picket fence (acting as a diffraction grating)! A world where we are waves (or objects, or both, or neither)?
Such musings are part of great stories “The Quantum Safari” and “Quantum Snooker” in a wonderful science fiction book “The NEW World of Mr Tompkins” (Authors George Gamow and Russell Stannard) published by Cambridge University Press. Challenge and delight yourself.
And there are a few other stories based on imagined values of the other universal constants. Such as a universe in which the speed of light is about 30 kilometres per hour.
SELF CHECK - Some thinking tasks
I was almost blinded by so many photons coming from the oncoming car.
Finding your way around .....
You can browse or search the Aha! Learning chemistry website in the following ways:
You can browse or search the Aha! Learning chemistry website in the following ways:
- Use the drop-down menus from the buttons at the top of each page to browse the modules chapter-by-chapter.
- Click to go to the TABLE OF CONTENTS (also from the NAVIGATION button) to see all available chapters and modules in numbered sequence.
- Click to go to the ALPHABETICAL INDEX. (also from the NAVIGATION button).
- Enter a word or phrase in the Search box at the top of each page.
